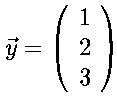LaTeX-Manual-Sekundarstufe2

Analysis

Schwarzschrift	Prosa	LaTeX	...
[math]\displaystyle{ n \to \infty }[/math]	n geht gegen unendlich	n \to \infty
[math]\displaystyle{ \lim_{h \to 0} }[/math]	Limes h gegen 0	\lim_{h \to 0}
[math]\displaystyle{ \lim_{x \to x_0} }[/math]	Limes x gegen x Index 0	\lim_{x \to x_0}
[math]\displaystyle{ f\ ' (x), f\ ''(x) }[/math]	f Strich von x, f zwei Strich von x	f'(x), f''(x)
[math]\displaystyle{ \sum_{i=0}^n A_n }[/math]	Summe von i gleich 0 bis n über A Index n	\sum_{i=0}^n A_n
[math]\displaystyle{ \int_a^b f(x) dx }[/math]	Integral von a bis b über f von x dx	\int_a^b f(x) dx

Stochastik

Schwarzschrift	Prosa	LaTeX	...
[math]\displaystyle{ n! }[/math]	n Fakultät	n!
[math]\displaystyle{ {n \choose k} }[/math]	Binomialkoeffizient n über k	{n \choose k}
[math]\displaystyle{ \sigma \Omega }[/math]	klein Sigma groß Omega	\sigma \Omega

Analytische Geometrie

Schwarzschrift	Prosa	LaTeX	...
[math]\displaystyle{ \vec{x} = (x \; y \; z) }[/math]	Vektor x ist gleich Zeilenvektor x y z Vektorende	\vec{x} = (x \; y \; z)	\vec{x} = (x & y & z)
	Vektor y ist gleich Spaltenvektor 1 2 3 Vektorende	\vec{y} = \mat{1 \\ 2 \\ 3}
	Matrix groß A ist gleich Zeilenanfang 1 2 3 Zeilenende Zeilenanfang 4 5 6 Zeilenende Matrixende	A = \mat{ 1 \; 2 \; 3 \\ 4 \; 5 \; 6 }	A = \mat{ 1 & 2 & 3 \\ 4 & 5 & 6 }

Anmerkungen

Anmerkung 1)

Diese Kurzschreibweise stammt aus der "Dresdener Abkürzungsliste" von U. Nitsch. Beim Übersetzen wird diese mit der Datei vorspann.tex automatisch eingebunden. Spaltenvektoren werden hier als Matrizen mit einer Spalte betrachtet.